Until Neil Bohr’s model, the only aspect of light that was examined was its wave character. Later, in his explanation of the quantum theory, Max Planck suggested that light is composed of tiny pockets of energy, which are made up of photons or quanta. It was then assumed that light has a particle nature and that each packet of light continuously emits a constant amount of energy.
The energy of photons can be represented by the equation:
E = hυ = h c / λ
Here, h is Plank’s constant
υ refers to the frequency of the waves
λ implies the wavelength of the pockets
As a result, this implies that light possesses both the property of particle duality as well as a wave.
The quantum theory of radiation proposed by Max Planck and Albert Einstein states that light has a dual nature, i.e., it can behave as both a particle and a wave under certain conditions.
De-Broglie claimed that the equation E = mc2 implies that a particle with mass “m” can be destroyed and turned into energy “E” of pure electromagnetic radiation. If electromagnetic radiation may have wave-particle duality, then the particle of mass “m” that was turned into radiation should have the same.
The explanation is that the simple conversion of a particle into radiation should not result in the latter developing new properties that were not present in the former. As a result, the well-established fact that electromagnetic radiation exhibits wave-particle duality suggests that particles such as electrons should also display wave-particle duality.
So, Louis V. de Broglie, a young French Ph.D. student, proposed in 1924 that if light were made up of particles and waves, electrons and other particles should be the same. The phenomenon is known as wave-particle duality.
Interesting Science Videos
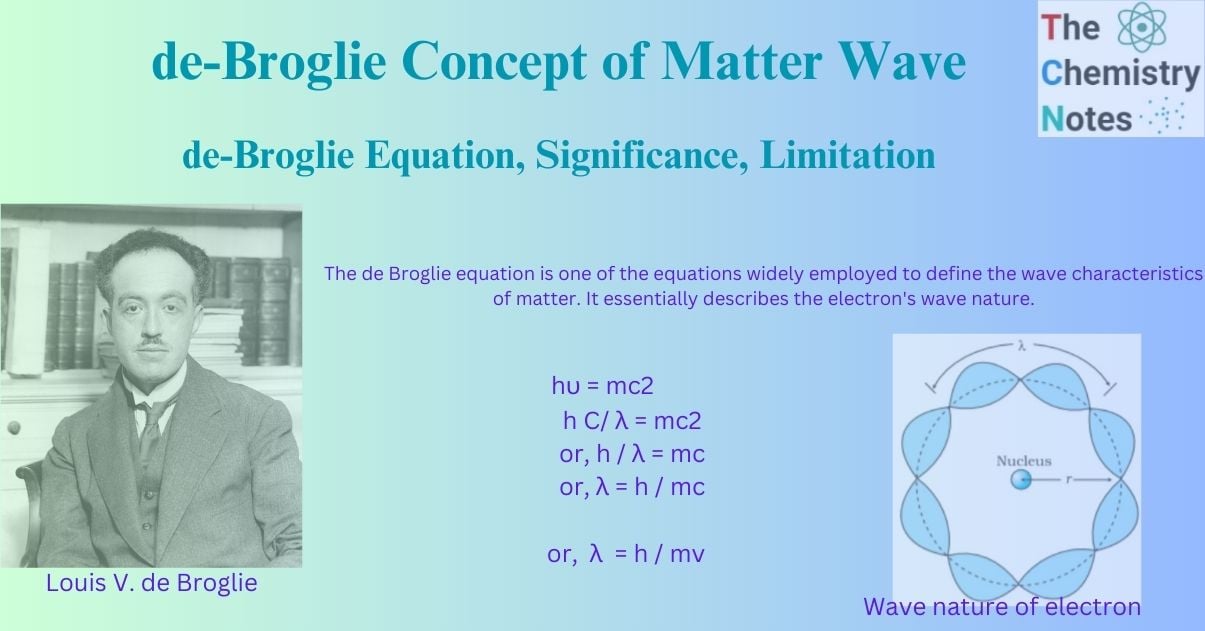
Wave nature of electron
According to Louis de-Broglie, all matter particles (electrons, protons, atoms, molecules, and so on) in motion have properties of both a matter particle and a wave. The wave character associated with a matter particle is known as the de-Broglie matter-wave. The revolutionary concept of wave-particle duality in matter led him to the crucial conclusion that since the electron is a matter particle that constantly rotates around the nucleus, it behaves both as a particle and a wave. This is referred to as the electron’s dual nature. However, it should be noted that the de-Broglie matter wave is not electromagnetic.
de-Broglie Equation
The de Broglie equation is one of the equations widely employed to define the wave characteristics of matter. It essentially describes the electron’s wave nature. The de-Broglie theory stated that matter particles in motion are related to wave character and may also be associated with other wave qualities such as wavelength, amplitude, and frequency. De-Broglie developed his idea with the help of Planck’s quantum theory and Einstein’s theory of relativity. He discovered a link between the magnitude of wavelength and the mass ‘m’ of a matter particle traveling at velocity ‘v’.
From Planck’s equation, the energy associated with a photon is given by,
E = hυ ………….(I)
h = Planck’s constant
υ = Frequency of radiation.
Einstein’s special theory of relativity (1905) has one of the consequences that a photon has the energy that can be expressed as,
E = mc2…………(II)
Where,
m = Mass of particle
c = Velocity of light
The famous equation expresses the relationship between mass and energy. The equation does not indicate that a photon has mass. It signifies that since a photon has energy its energy is equivalent to some mass.
Now,
Equating equation (i) and (ii), we get,
or, hυ = mc2…………(III)
Since, υ = C/ λ
Equation (III) becomes
h C/ λ = mc2
or, h / λ = mc
or, λ = h / mc
This equation represents the wavelength of radiation. A similar equation can be applied to a matter particle by substituting the velocity of that particle for the velocity of light. Then the equation can be written as:
or, λ = h / mv
= h/p ……….(IV)
Where, m = mass of particle.
V = velocity of the particle
Λ = wavelength of matter wave.
P = Momentum of the particle.
This equation is called the de-Broglie equation and wavelength is referred to as de-Broglie wavelength. If mass and velocity are given, this equation can be used to calculate the wavelength of a moving particle.
Significance of de-Broglie Equation
The de Broglie hypothesis established that wave-particle duality was a fundamental principle shared by both radiation and matter, rather than an aberrant property of light. As a result, wave equations can be utilized to describe material behavior if the de Broglie wavelength is properly applied.
De Broglie waves around a closed loop, such as those associated with electrons orbiting nuclei in atoms, can continue only if the standing waves fit equally around the circle; otherwise, they cancel out. Due to this restriction, electrons in atoms choose only specific configurations.
This equation can be used for the prediction of the wavelength of the matter-wave.
De Broglie waves explain the arrival of subatomic particles at previously unanticipated sites because their waves permeate boundaries in the same way that sound does. As a result, a heavy atomic nucleus might occasionally eject a fragment of itself in a process known as alpha decay. As a particle, the fragment of the nucleus (alpha particle) lacks the energy to overcome the force barrier encircling the nucleus; nevertheless, as a wave, it may pass through the barrier—that is, it has a finite probability of being located outside the nucleus.
Limitation of the de-Broglie equation
The de-Broglie equation holds for all particles, however, it is only significant for small particles, such as huge particles in motion, which possess wavelength but is neither measurable nor observable. Let us compute the wavelengths of large and small particles and compare their results.
The de-Broglie wavelength for large particle
Let us suppose a ball having a mass of 1 kg is moving with a velocity of 10 m/s.
Then from de-Broglie equation,
Wavelength (λ) = h / mv = 6.626 x 10-34 / 1 x 10
= 6.626 x 10-35 m
It is too small to measure by the instrument. Hence, this equation is not significant for large particles.
The de-Broglie wavelength for small particles (e.g: electrons)
Let us suppose an electron having a mass of 9.109 x 10-31 kg is moving with a velocity of 2.1 x 106 m/s.
Then from de-Broglie equation,
Wavelength (λ) = h / mv = 6.626 x 10-34 / 9.109 x 10-31 x 2.1 x 106
= 0.346 x 10-9 m
This wavelength is quite close to the wavelength of X-rays and so measurable. As a result, this equation is only relevant for small particles in motion.
References
- Lee J. D. (1977). A new concise inorganic chemistry (3d ed.). Van Nostrand Reinhold. Retrieved May 26 2023 from https://archive.org/details/newconciseinorga00leej.
- Concept of modern physics by Arthur Beiser.
- https://www.vedantu.com/iit-jee/de-broglie-equation.
- http://physics.unm.edu/Courses/Fields/Phys2310/Lectures/lecture35.pdf.
- https://byjus.com/jee/de-broglie-equation/.
- https://unacademy.com/content/neet-ug/study-material/chemistry/de-broglie-principle-and-hypothesis/
