When a solute is added, the boiling point of a solvent rises, a process known as elevation of boiling point. The boiling point of a solvent solution that contains a non-volatile solute is greater than the boiling point of the solvent alone. For example, the boiling point of a solution of sodium chloride (salt) and water is higher than that of pure water.
It depends on the solute-to-solvent ratio but not the solute’s identity since boiling point elevation is a colligative property of matter. This suggests that the amount of solute added to a solution affects how high its boiling point rises. The boiling point will rise according to the solute concentration in the solution.

Interesting Science Videos
Elevation of Boiling point: Why Does It Occur?
The temperature at which a liquid’s vapour pressure equals the atmospheric pressure is known as the boiling point of the substance. Non-volatile substances have very low vapour pressures (assumed to be zero) and do not readily evaporate. The vapour pressure of the resultant solution is lower than the vapour pressure of the pure solvent when a non-volatile solute is added to it.
The solution must thus be exposed to more heat in order for it to boil. The elevation of boiling point is shown by the rise in the solution’s boiling point. The vapor pressure of the solution continues to fall and the boiling point of the solution continues to rise as the concentration of the additional solute increases.
Equation for Boiling Point Elevation
Let Tb be the boiling point of a pure solvent and that of solution be solution be T. Thus, the difference between these two temperatures is called elevation of boiling point.
Elevation of Boiling Point (∆T) = T – Tb
Figure shows variation of vapor pressure with the temperature of pure solvent and solution 1 and solution 2. As vapor pressure of solution is lower than that of a pure solvent, vapor pressure curve of pure solvent lies above the curve of solution.

In the figure, let P, P1, and P2 be the vapor pressures of pure solvent, solution 1, and solution 2 respectively at the boiling points of pure solvent, Tb. The boiling points of solution 1 and 2 are T1 and T2 respectively.
For very dilute solution, vapor pressure curve lines are almost parallel straight lines.
Therefore for similar triangles ACE and ABD, we have
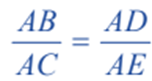
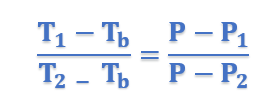
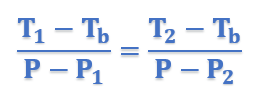
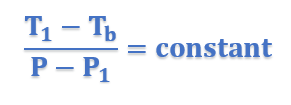
In general form, above equation can be written as;
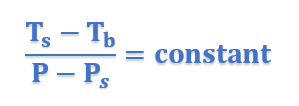

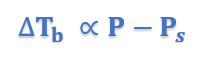
This equation thus proves that elevation of boiling point is directly proportional to lowering of vapor pressure.
Where,
Ts =Boiling Point of Solution
Ps = Vapor Pressure of Solution
Tb = Boiling point of Pure Solvent
P = Vapor Pressure of Pure Solvent
∆Tb = Ts – Tb [∆Tb = Elevation of Boiling Point]
P – Ps = Lowering of Vapor Pressure
Determination of Molecular Weight of Solute by Elevation of Boiling Point
Since P is constant for the same solvent at a fixed temperature, we can write

But from Raoult’s Law for dilute solutions,

Since M (mol mass of solvent) is constant

From equation (1) and (3);
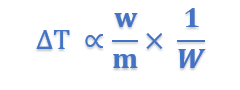
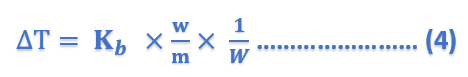
where Kb is a constant called Boiling point constant or Ebulioscopic constant of molal elevation constant.
If w/m = 1, W = 1, Kb = ΔT
Thus, Molal elevation constant may be defined as the boiling-point elevation produced when 1 mole of solute is dissolved in one kg (1000 g) of the solvent. If the mass of the solvent (W) is given in grams, it has to be converted into kilograms. Thus the expression (4) can be written as;
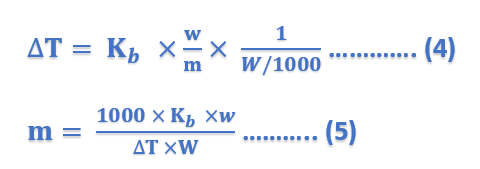
where ΔT = elevation of boiling point; Kb = molal elevation constant; w = mass of solute in grams; m = mol mass of solute; and W = mass of solvent in grams.
Sometimes the value of Kb is given in K per 0.1 kg (100 g). In that case, the expression (5) becomes
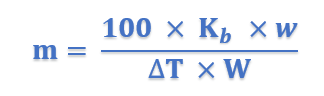
The value of Kb: The value of Kb can be determined by measurement of ΔT by taking a solute of known molecular mass (m) and substituting the values in expression (5).
Units of Kb: From equation (4), we have;
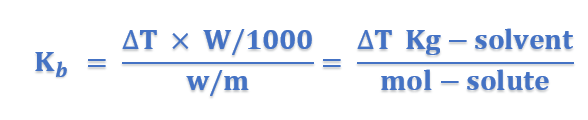
Thus the units of Kb are;

The constant Kb, which is characteristic of a particular solvent used, can also be calculated from thermodynamically derived relationship;
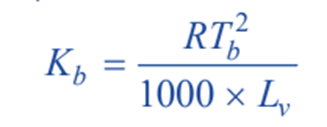
where;
R = gas constant;
Tb = boiling point of solvent;
Lv = molar latent heat of vaporization.
Thus for water R = 8.134 J mol–1; T = 373 K : Lv= 2260 J g–1
Therefore,
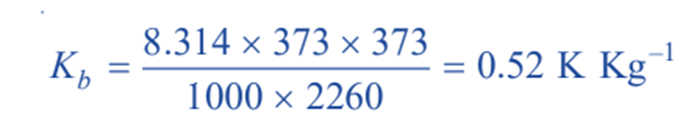
The Molal boiling point constant for some common solvents
| Solvent | Kb per kg (1000 g) | Kb per 0.1 kg (100 g) |
| Water | 0.52 | 5.2 |
| Propanone (acetone) | 1.70 | 17.0 |
| Ethoxyethane (ether) | 2.16 | 21.6 |
| Ethanoic acid (acetic acid) | 3.07 | 30.7 |
| Ethanol | 1.75 | 17.5 |
Measurement of Elevation of Boiling Point
The elevation of boiling point can be measured using a variety of techniques. Some of these are as follows:
Landsberger-Walker Method
This method was developed by Landsberger and modified by Walker. The equipment needed for this procedure consists:
(i) An inner tube with a hole in its side and graduated in ml;
(ii) A boiling flask which sends solvent vapour in to the graduated tube through a ‘rosehead’ (a bulb with several holes)
(iii) An outer tube which receives hot solvent vapour issuing from the side-hole of the inner tube;
(iv) A thermometer reading to 0.01 K, dipping in solvent or solution in the inner tube

Procedure:
The graduated tube is filled with pure solvent, and vapor from the same solvent that is boiling in a different flask is then transferred into it. The solvent in the tube boils as a result of the vapor’s latent heat of condensation. When the solvent starts boiling and the temperature becomes constant, its boiling point is noted.
The supply of vapour is now temporarily cut off, and a weighted pellet of the solute is dropped into the solvent in the inner tube. The solvent vapour is passed through again until the boiling point of the solution is attained and recorded. After stopping the solvent vapor, the solution’s volume is measured and the thermometer and rosehead are lifted out of the solution.
From a difference in the boiling points of solvent and solution, we can find the molecular weight of the solute by using the expression;
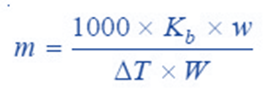
where;
w = weight of solute taken,
W = weight of solvent which is given by the volume of solvent (or solution) measured in ml multiplied by the density of the solvent at its boiling point.
Cottrell’s Method
Cottrell (1910) developed a technique that was superior to the Landsberger-Walker technique.
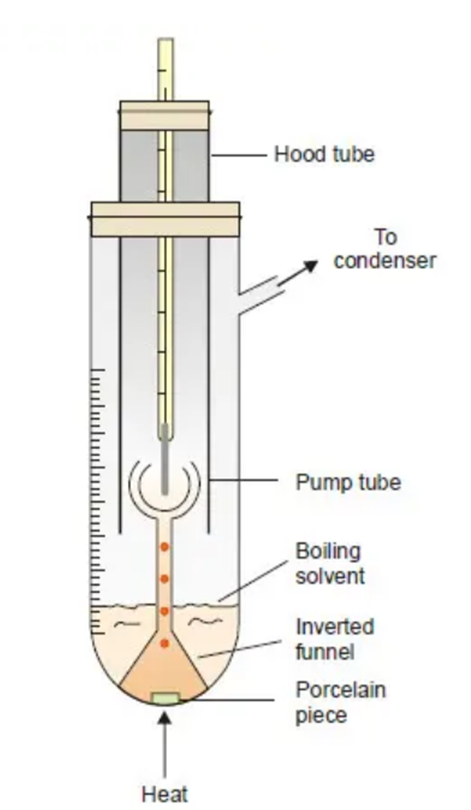
Apparatus Required:
(i) a graduated boiling tube containing solvent or solution;
(ii) a reflux condenser which returns the vapourised solvent to the boiling tube;
(iii) a thermometer reading to 0.01 K, enclosed in a glass hood;
(iv) A small inverted funnel with a narrow stem which branches into three jets projecting at the thermometer bulb.
Beckmann Thermometer: It is differential thermometer. It is designed to measure
small changes in temperature and not the temperature itself.
Procedure:
- The equipment is set up as shown in Figure above
- A porcelain piece is positioned in the boiling tube with solvent. It is heated with a tiny flame (micro burner).
- As soon as the solution begins to boil, solvent vapour that emerges from the porcelain object pumps the boiling liquid into the stem. As a result, a constant stream of boiling liquid and solvent vapour is released around the thermometer bulb.
- The temperature becomes constant and the boiling point of the pure solvent is recorded.
- Next, a weighted pellet of the solute is introduced to the solvent, and when the temperature becomes constant, the boiling point of the solution is reported. Additionally, the boiling tube’s solution volume is noted.
- The rise of the boiling point is caused by the difference between the boiling points of the solvent and the solute.
- The volume of the solution is transformed into mass when determining the solute’s molecular weight by multiplying it by the solvent’s density at its boiling point.
References
- Atkins, Peter and de Paula, Julio. Physical Chemistry for the Life Sciences. New York, N.Y.: W. H. Freeman Company, 2006. (124-136).
- Laidler, K.J.; Meiser, J.L. (1982). Physical Chemistry. Benjamin/Cummings. ISBN 978-0618123414
- https://byjus.com/chemistry/elevation-boiling-point/#:~:text=What%20is%20Boiling%20Point%20Elevation,that%20of%20the%20pure%20solvent.
- https://byjus.com/chemistry/elevation-boiling-point/
- T. Engel and P. Reid, Physical Chemistry (Pearson Benjamin Cummings 2006
- Tro, Nivaldo J. (2018). Chemistry: Structure and Properties (2nd ed.). Pearson Education. ISBN 978-0-134-52822-9.
- McQuarrie, Donald, et al. Colligative properties of Solutions” General Chemistry Mill Valley: Library of Congress, 2011. ISBN 978-1-89138-960-3.
