A compound’s concentrations in a mixture of two immiscible solvents at equilibrium are expressed as a partition coefficient (P) or distribution coefficient (D).
Interesting Science Videos
What is Partition Coefficient?
The ratio of a compound’s unionized species concentrations in a mixture of two immiscible phases is known as the partition coefficient.
This phenomenon is also commonly referred to as “P.” To calculate the two-phase system’s partition coefficient, the two phases must be in equilibrium with one another. The solubility of each unionized species in this mixture is quantified by this ratio.
Understanding the definitions of solutes, solvents, solutions, and solubility is thus required to calculate a partition coefficient.
- A solution is a homogeneous mixture of a solute and a solvent.
- The solute is the substance that dissolves in the solvent.
- Solubility refers to the degree to which a solute dissolves in a solvent.
When calculating the P, we frequently take hydrophilic-hydrophobic systems into account. This also serves as a gauge of the solute’s hydrophobicity or lipophilicity, respectively. Further this phenomenon is crucial in figuring out how drugs are distributed throughout our bodies.
Partitioning is based on Nernst’s Law of Distribution. Nernst investigated solute partitioning using pairwise solvent combinations and discovered that the distribution of a solute can be described by the ratio of the solute within the two liquids.
Partition Coefficient Equation
The formula equals the ratio of solute’s concentration when it is stationary to the solute’s concentration when it shows mobility. It is proportional to the amount of stable solute and inversely proportional to the amount of moving solute. It has the symbol Kd and is a unitless quantity because it is the ratio of two identical quantities.
Kd = Cs/Cm
where,
Kd is the partition coefficient,
Cs is the concentration of solute in stationary phase,
Cm is the concentration of solute in mobility phase
Determination of Partition Coefficient
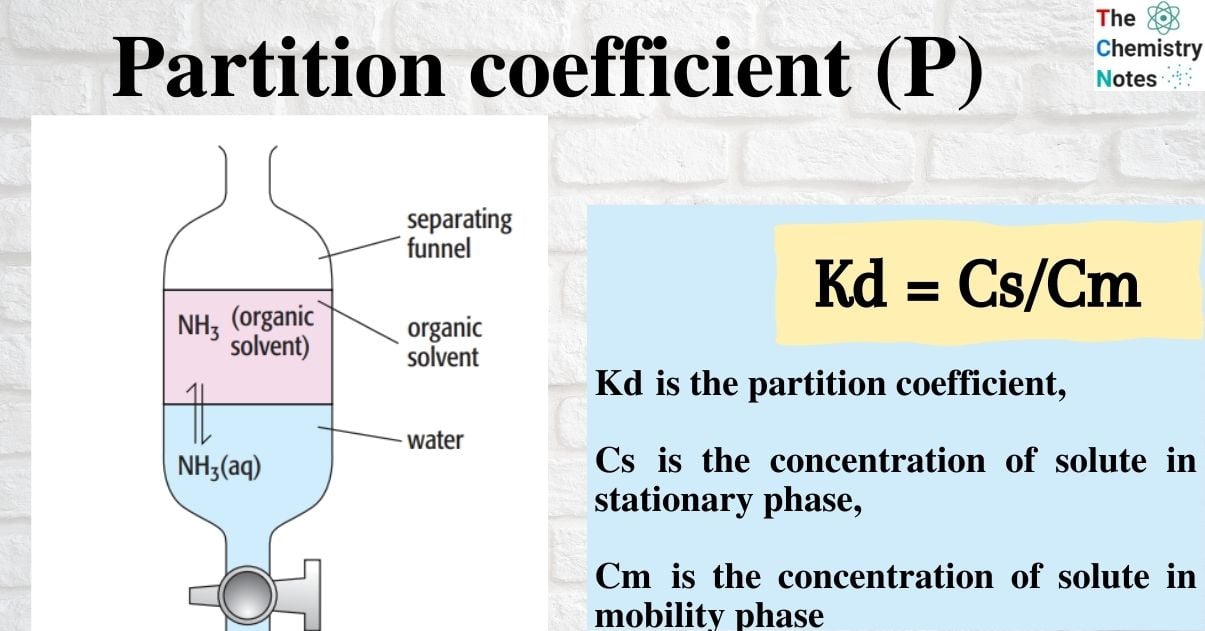
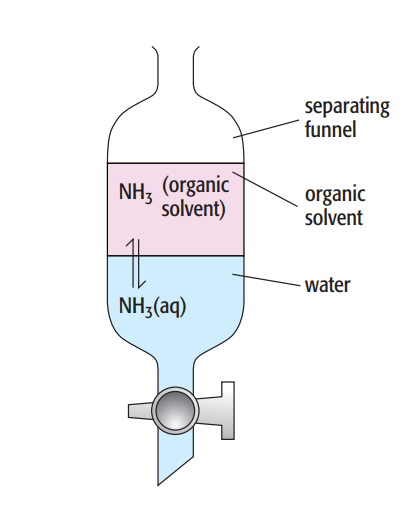
Consider ammonia dissolved in two immiscible solvents, or solvents that do not mix and instead form two distinct layers.
Distribution Coefficient (D)
The distribution coefficient measures the ratio of a compound’s ionized and non-ionized species in a solution of two immiscible phases.
This phenomenon can be designated as “D.” Here, water or an aqueous solution is essentially one of the two immiscible phases. Typically, the other phase is a hydrophobic phase that is inimical to water (or any other aqueous phase we use here). Because it is such a small value, we typically express it as a logarithmic value.
The organic solvent and an aqueous ammonia solution are shaken in a separating funnel. Since the ammonia is soluble in both solvents, a dynamic equilibrium is created when the mixture is allowed to settle. At this point, ammonia molecules are transitioning from the organic layer to the aqueous layer at the same rate as they are doing the reverse:
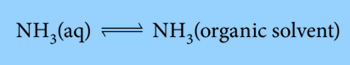
The partition coefficient (P), also known as the equilibrium constant, can be calculated. The equilibrium constant that connects the concentration of a solute partitioned between two immiscible solvents at a specific temperature is thus known as the partition coefficient.
Applications
- The ease with which a drug can reach its intended target in the body, the strength of the effect it will have once it gets there, and how long it will stay in the body in an active form are all significantly influenced by the drug’s distribution coefficient.
- The distribution coefficient has a significant impact on the drug’s ADME properties in the context of pharmacokinetics, which refers to how the body assimilates, metabolizes, and excretes a drug. Therefore, a compound’s hydrophobicity (as determined by its distribution coefficient) is a key factor in determining how drug-like it is.
- Partition coefficient can also be used to predict the mobility of radionuclides in groundwater
- The octanol-water partition coefficient (Kow ) is used in hydrogeology to predict and model the migration of dissolved hydrophobic organic compounds in soil and groundwater.
- The distribution of various impurities between molten and solidified metal in metallurgy is largely influenced by the partition coefficient. Hence it is a crucial factor in zone melting purification. Because it determines how well an impurity can be eliminated through directional solidification, which is modeled by the Scheil equation.
References
- Kwon Y (2001). “4.2.4: Partition and Distribution Coefficients”. Handbook of Essential Pharmacokinetics, Pharmacodynamics and Drug Metabolism for Industrial Scientists.
- Sangster J (1997). Octanol–Water Partition Coefficients: Fundamentals and Physical Chemistry. Wiley Series in Solution Chemistry. Vol. 2. Chichester: John Wiley & Sons Ltd
- Leo A, Hansch C, and Elkins D (1971). “Partition coefficients and their uses”. (secondary). Chem Rev. 71
- https://en.wikipedia.org/wiki/Partition_coefficient
- https://study.com/learn/lesson/partition-coefficient-concept-equation.html
- https://www.bionity.com/en/encyclopedia/Partition_coefficient.html#Applications
