Ideal Gas Equation: Ideal Gas and Laws

The following physical characteristics define the gaseous state:

Gases have a high compressibility.
Gases apply pressure in all directions equally.
Compared to solids and liquids, gases are significantly less dense.
The volume and shape of gases are not constant. These presuming the container’s volume and shape.
Without using any mechanical assistance, gases combine perfectly and evenly in all ratios.
Gases are simple because there are very little forces between their molecules.

Table of Contents

Interesting Science Videos

The kinetic theory of gases

The kinetic theory of gases describes the notion that molecules in gases are constantly in motion. The underlying assumptions of this theory are:

Gas molecules move rapidly and randomly.
The distance between the gas molecules is much greater than the diameter of the molecules so the volume of the molecules is negligible.
There are no forces of attraction or repulsion between the molecules.
All collisions between particles are elastic – this means no kinetic energy is lost in collisions (kinetic energy is the energy associated with moving particles
The temperature of the gas is related to the average kinetic energy of the molecules.

An ideal gas is a hypothetical gas that meets this criteria. In reality, the gases we encounter do not exactly fit this description, though they may come close.
Real gases are the gases that we come into contact with. The behavior of noble gases with small atoms, like helium and neon, is close to that of an ideal gas caused by the negligibly small intermolecular forces.

Real Gases and Ideal Gases

Real gases are non-ideal gases because real gases’ molecules occupy space and interact, contravening the ideal gas law.

The fact that real gases deviate from the ideal gas law is due to these intermolecular interactions between the gas particles. Real gases can behave differently from how they should, especially under extreme pressure and temperature conditions. The compressibility factor is used to calculate the magnitude of the deviation. By dividing the product of pressure and volume by the product of the gas constant and temperature (PV/RT) for one mole of a given substance, one can find the compressibility factor by solving for n in the ideal gas law. This ratio of PV to RT should be exactly equal to one under ideal circumstances.

An ideal gas is one in which there are no intermolecular attractive forces and all collisions between atoms or molecules are perfectly elastic.

It can be imagined as a group of perfectly hard spheres that collide but do not otherwise interact with one another. Any change in internal energy in such a gas results in a change in temperature because all of the internal energy is present in the form of kinetic energy.

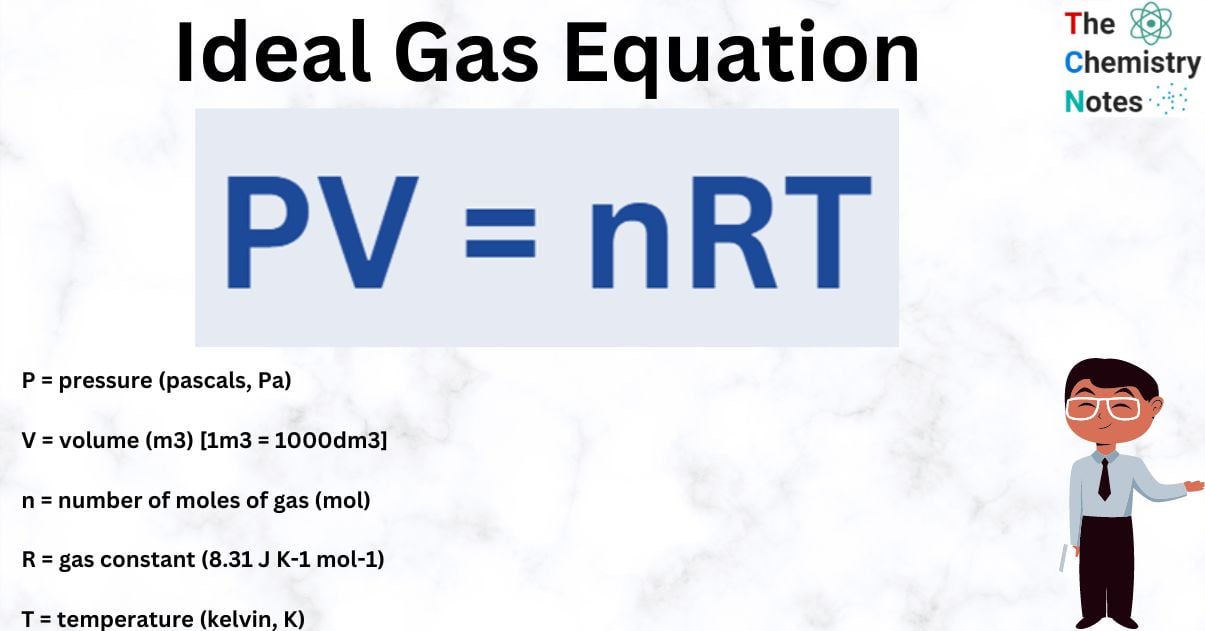
Ideal Gas Equation

The ideal gas equation describes the relationship between an ideal gas’s pressure, volume, temperature, and number of moles of gas:

PV = nRT

Where;

P = pressure (pascals, Pa)

V = volume (m³) [1m³ = 1000dm³]

n = number of moles of gas (mol)

R = gas constant (8.31 J K^-1 mol^-1)

T = temperature (kelvin, K)

The ideal gas laws limitations

To demonstrate how the volumes of gases change with temperature and pressure, scientists have made precise measurements. These demonstrate to us that gases frequently behave differently from how we would anticipate an ideal gas to. This is due to the following reason why real gases don’t always follow the kinetic theory:

We cannot ignore the volume of the molecules themselves because there isn’t zero attraction between them.

At very high pressures and very low temperatures, these differences stand out in particular:

The molecules are close to each other
The volume of the molecules is not negligible compared with the volume of the container
There are van der Waals’ or dipole–dipole forces of attraction between the molecules
Attractive forces pull the molecules towards each other and away from the walls of the container
The pressure is lower than expected for an ideal gas
The effective volume of the gas is smaller than expected for an ideal gas.

Calculations using the ideal gas equation

We can calculate the fifth physical quantity in the general gas equation if we know any four of the five physical quantities.

Determine the volume that 0.500 mol of carbon dioxide occupies at 19°C and 150kPa of pressure. [R = 8.31 J K^-1 Mol^-1]

Step 1: Convert temperature and pressure to the proper units.

150 kPa = 150 * 1000 Pa = 150000Pa;
19°C = 19 + 273 = 292K

Step 2: Rearrange the general gas equation into the desired format.

PV = nRT
V = (nRT)/V

And now Substitute the numbers.

V = (0.500 × 8.31 × 292) / 150000
V = 8.09dm³

Calculating relative molecular masses using ideal gas equation

Using a mass spectrometer is a reliable way to determine a substance’s relative molecular mass. However, the general gas equation can be used to determine the mass of gas in a large flask, though it is a less precise method. We can find the relative molecular mass of a gas by simply substituting it into the general gas equation because the number of moles is equal to the mass of a substance divided by its relative molecular mass.

A flask of volume 2.0 dm³was found to contain 5.28 g of a gas. The flask had a 200 kPa pressure and a 20 °C temperature. Determine the gas’s relative molecular mass.
R = 8.31 J K^-1Mol^-1

Step 1: Convert pressure, temperature, and volume into the proper units, then determine how many moles of oxygen are present.

200 kPa = 2.00 × 10⁵ Pa
20°C = 20 + 273 = 293 K
200 dm³ = 2.00 × 10^–3 m³

Step 2: Rearrange the general gas equation into the required form.

PV = nRT Also; n = m/M_r
PV = (m/M_r) RT
M_r = (mRT) / PV