Viscosity is a measure of the resistance to flow that a liquid exhibits because of the internal friction that occurs between the different layers of the fluid. The majority of fluids have some resistance to motion, and we refer to this resistance as “viscosity.” The relative motion that occurs between the different layers of the fluid is what gives rise to the property known as viscosity. To be more specific, it measures the resistance to flow that develops as a result of the internal friction that occurs between the fluid layers as they move past one another when fluid is moving. Viscosity is a property of a fluid that can be regarded as a measurement of its thickness or its resistance to the movement of objects through the fluid.
Simply, molecular layers can be thought of as being stacked one on top of the other in a liquid, giving the appearance of the liquid having a layered structure. When a shear force is given to a liquid, it causes the liquid to flow. On the other hand, the forces of friction that exist between the layers act as a barrier to this flow. A liquid’s viscosity can be thought of as a measurement of the resistance to the flow of liquid. The viscosity of a liquid is one of the distinguishing properties of the liquid. It is recognized that a liquid is more viscous than others when two liquids are moved at the same velocity but one liquid is more difficult to move. Fast-moving liquids exhibit less resistance, which indicates that they are less viscous, whereas slower-moving liquids exhibit greater resistance, which indicates that they are more viscous.
Interesting Science Videos
What is viscosity?
The resistance to the flow of liquid is called viscosity. The interaction between molecules in a fluid gives rise to its viscosity i.e. when the individual layers of a liquid are moving with respect to one another, we observe viscosity. Most of this liquid is not ideal, and it acts as a barrier or offers resistance to movement. The energy needed to make a fluid flow is determined by its viscosity, which is analogous to friction between moving solids. A high viscosity indicates that the fluid is resistant to motion due to the high internal friction caused by the strong intermolecular tensions between its layers. Instead, the molecular composition of low-viscosity fluid results in minimal friction during motion, making the fluid easy to move.
Increasing the temperature causes a rise in the viscosity of gases, while the viscosity of liquids drops fast. Therefore, liquids have better viscosity at higher temperatures while gases have a lower and hence move slowly. In addition, viscosity is an intensive property since it is independent of the amount of matter changes.
In order to acquire a greater understanding of the concept of viscosity, let’s take into account the movement of liquid through a pipe. As a fluid moves through a pipe, one can imagine a number of distinct layers forming in a concentric pattern. The concentric layers that are closest to the pipe’s wall travel more slowly, in contrast to the concentric layers that are located in the pipe’s core, which move faster. The speeds of many concentric layers of liquid inside a tube continue to increase as they go closer and closer to the tube’s center from the tube’s outside wall.

The layer of liquid closest to the tube wall is nearly stationary, and the speed of each successive layer increases steadily moving toward the tube’s center. The velocity of the liquid is greatest in the central layer. In this scenario, the layer of liquid traveling more slowly slows down the layer of liquid moving more quickly next to it. The term “viscosity” is used to describe this slowing/retarding effect. Let dx denote the separation between two layers and dv the velocity difference between them. This means that the gradient in velocity is dv / dx.
According to Newton, the viscous force depends on the following factors:
- Directly proportional to the cross-sectional area i.e. F ∝ A
- Directly proportional to the velocity gradient between the concentric layers i.e. F ∝ (dv / dx)
- Thus, F ∝ A (dv / dx)
- or, F= η A ( dv / dx)
- where η is a constant known as the coefficient of viscosity. Assuming dx = 1, A = 1 sq cm, and dv = 1 cm/sec, we have F = η. The coefficient of viscosity can be defined as the frictional force needed to keep a 1 cm/sec velocity difference between two parallel layers, each of which has an area of 1 sq cm.
Unit of Viscosity
In the CGS system, the unit of coefficient of viscosity is dyne.sec.cm-2 which is also called poise.
In S.I. system, η = Netwon (N)/m2 = m/m.sec-1 = N sec m-2
What is Fluidity?
The reciprocal of viscosity is called fluidity.
Types of Viscosity
- Dynamic Viscosity: When measuring force directly gives an indication of viscosity. It is calculated as the shear stress in a material divided by the shear strain caused by an external force. Liquid flow velocity can be determined using dynamic viscosity.
- Kinematic Viscosity: Force plays no role in kinematic viscosity. It is the dynamic viscosity of the fluid divided by its density. To calculate it, divide the fluid’s dynamic viscosity by the fluid’s mass density. Compared to dynamic viscosity, kinematic viscosity is more informative.
- Laminar flow: By definition, fluids that travel smoothly or in a regular course from one layer to the next are said to be in laminar flow. Lower velocities characterize the laminar flow. When there is no friction between the layers, the fluid will flow in parallel sheets, a phenomenon known as laminar flow.
Measurement of Viscosity
Pioseulle’s equation can be used to calculate a liquid’s viscosity, which is a measure of the substance’s resistance to flow. This expression, which dictates the movement of a liquid as it passes through a capillary, can be written as follows:
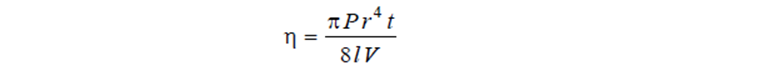
where V is the volume of the liquid that is passing through the capillary at time t, P is the pressure head, r is the radius of the tube, and l is the length of the tube. There is a significant amount of challenge involved in the experimental measurement of P, r, l, and V. As a consequence of this, it is not possible to derive the absolute viscosity coefficient (η) directly from Poiseulle’s equation.
When determining the viscosity of a liquid, it is common practice to compare it to the viscosity of water. This property is referred to as the Relative Viscosity. Let’s say that t1 and t2 represent the times that a predetermined volume (V) of each of the liquids flows through the same capillary. The above Equation can be used to get the term for relative viscosity, which is written as (η1/ η2).

Since the pressure head is proportional to the density (d) of the liquid, the equation becomes:

Substituting the value of the viscosity coefficient of water (η2) in (3), we can find the absolute viscosity of the given liquid (η1).
Determination of viscosity of liquid using Ostwald Viscometer
The Ostwald viscosimeter, often known as a viscometer, is an apparatus that is most frequently utilized for the purpose of determining the relative viscosity of a liquid. The figure given below illustrates a simple example of it. The left-hand limb is essentially a pipette, and it has two calibration marks on it. These marks are labeled A and B. The pipette is connected to bulb C in the right-hand limb through a short segment of a capillary tube.
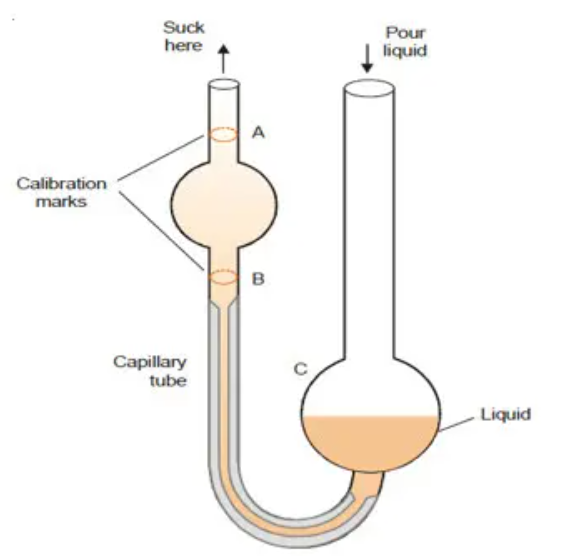
Ostwald Viscometer
image source: https://readchemistry.com/2022/08/23/viscosity-measurement-of-viscosity/
A measured amount of liquid, let’s say 25 milliliters, is transferred into bulb C using a pipette. With the assistance of a rubber tubing that is attached to the left arm, the liquid is sucked up close to the top of the limb. After that, the liquid is allowed to flow freely once more into bulb C. A stopwatch is used to record the amount of time (t1) that is spent traveling from A to B. After that, the instrument is cleaned, and the experiment is performed again, this time using water of approximately the same volume. The time that it takes water to flow from point A to point B, denoted by t2, is recorded. A pyknometer is used to determine the density of the liquid (denoted by d) as well as the density of water (denoted by dw). The expression that is used to determine the relative viscosity coefficient is as follows:
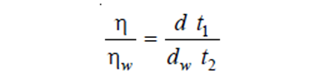
Knowing the value of the viscosity coefficient of water (ηw) at the temperature of the experiment, the absolute viscosity coefficient (η) of the given liquid can be found.
Suggested video
Newtonian and Non-Newtonian Fluid Viscosity
A constant viscosity is characteristic of the vast majority of fluids, which are collectively referred to as Newtonian fluids. There is a proportional but consistent rise in the amount of resistance experienced when the force that is being applied is increased. No matter how much force is applied to a Newtonian fluid, it will continue to behave in a manner consistent with that of a fluid.
Non-Newtonian fluids, on the other hand, do not have a constant viscosity; rather, it is highly variable and is determined by the force that is applied to the fluid. When subjected to a significant amount of force, Oobleck, also known as “slime” and frequently created in elementary school science classes, demonstrates behavior more akin to that of a solid than that of a Newtonian fluid. This is a prime example of a non-Newtonian fluid. Magnetorheological fluids are yet another category of non-Newtonian fluids that can be found in nature. These react to magnetic fields by almost completely solidifying, but when removed from the magnetic field, they revert to their liquid condition.
Factors affecting viscosity
Intermolecular attraction in liquid gives rise to the fraction between two layers flowing at different velocities. When it comes to gases, viscosity decreases as temperature rises, but the opposite is true for liquids. This is because “viscosity” has distinct meanings when used for liquids and gases.
- Unless the pressure is extremely low, the exchange of momentum between two moving layers is what causes the gas to be viscous.
- In liquid, it increases with an increase in pressure.
The viscosity of a fluid can be affected by a number of variables. These include:
- Fluid Temperature: Typically, as temperatures rise, liquids become less viscous. On the contrary, when temperature rises, gases become more viscous.
- Flow Conditions: The liquid’s viscosity is constant for laminar flow but varies for turbulent flow.
- Pressure: Gases tend to become more viscous as pressure is increased. The fact that liquids are incompressible has negligible consequences.
- Multiphase flow: Multiphase flows have a different overall viscosity depending on the volumes of the individual phases.
- Suspended particles: Suspended materials increases viscosity
Significance of Viscosity
The viscosity of a fluid is inversely proportional to its fluidity, which is defined as the ease with which a fluid can flow. It is, in simple terms, the friction that occurs within the fluid itself between the molecules that make up the fluid. In applications such as injection molding, spraying, and surface coating, viscosity plays a significant role since it affects the flow of the liquid being used. Other applications that benefit from this property include fluid transportation and lubrication engineering. To accurately forecast the behavior of a fluid, it is critical to have accurate viscosity measurements. For instance, if the tomato ketchup that is contained within the tube does not have the appropriate viscosity, the ketchup might not flow from the tube at all, or it might flow excessively.
Effect of Temperature on Viscosity
When the temperature is raised, the average kinetic energy of the molecules also rises, which has the effect of reducing the attractive force that exists between the molecules. It leads to a reduction in the viscosity coefficient. An empirical equation was provided by Arrhenius and Guzman that illustrates the relationship between temperature and the coefficient of viscosity.
η = A eEa/RT
Where, η = coefficient of viscosity, A= constant, Ea = Activation energy, R = universal gas constant, and T = absolute temperature
The viscosity of a liquid will drop as the temperature rises, but the viscosity of a gas will increase. To put it another way, the viscosity of a liquid will decrease as the temperature rises, whereas the viscosity of gases will increase as the temperature rises.
Why does water flow faster than honey?
In comparison to water, the viscosity of honey is significantly greater. In regard to the fact, it is significantly more viscous than water. When compared to water, honey has a higher cohesion, which is the measure of how strongly the particles in a fluid attract one another. Honey will flow more slowly than water because water has less cohesion and lower viscosity than honey. As a result, honey will take longer to flow than water.
Application of Viscosity
- Cooking: The viscosity of a liquid is an important factor to consider while preparing and serving food. When heated, cooking oils might or might not see a change in viscosity, although almost all of them become significantly more viscous after being cooled. When they are cooled, fats, which remain liquid at room temperature, take on a solid consistency. The consistency of sauces, soups, and stews is crucial to the preparation of a variety of cuisines. For instance, a thick potato and leek soup can be transformed into a French vichyssoise by simply reducing its viscosity. Honey, which is highly viscous and can alter the “mouth feel” of a dish, is an example of one of the fluids that can give food a different texture.
- Manufacturing: Lubrication is essential for the efficient operation of manufacturing machinery. Pipes can become clogged and jammed if lubricants are excessively thick. Thin lubricants don’t offer enough safety for moving parts.
- Lubrication in vechicles: It’s important to consider the oil’s viscosity before putting it in your vehicle. This is due to the fact that viscosity influences friction, and friction influences heat. The rate of oil consumption and the ease with which your vehicle will start in either hot or cold circumstances are also affected by viscosity. If your oil has a low viscosity index, it may get thinner as it heats, which could present issues as you drive on a hot summer’s day if you use it in your vehicle.
- Medicine: When fluids are administered intravenously, viscosity can play a crucial role in medicine. Too thick blood can cause life-threatening clots on the inside, while too thin blood won’t clot at all, so finding a balance between the two is crucial.
- The extent of a cohesive force, the strength of hydrogen bonds, and the boiling point may all be determined from the viscosity coefficient if one is familiar with its value.
References
- Halliday, David; Resnick, Robert; Krane, Kenneth S. (2010-04-20). Physics, Volume 2. John Wiley & Sons. p. 342.
- Arun Bahl, B. S. Bahl & G. D. Tuli, Essentials of Physical Chemistry, S. Chand and Company Ltd., New Delhi, 2012.
- Petrucci, et al. General Chemistry: Principles & Modern Applications. 9th ed. Upper Saddle River, New Jersey: Pearson/Prentice Hall, 2007.
- Natarajan, G, and Viswanath, D. Data Book On The Viscosity of Liquids. United States of America: Hemisphere Publishing Corporation, 1989.
